Eight Queens

Eight Queens

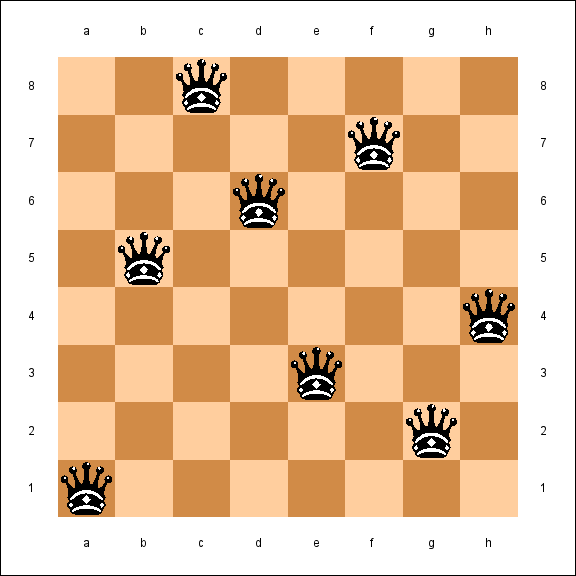
This is a classical problem in computer science. The objective is to place eight queens on a chessboard so that no two queens are attacking each other; i.e., no two queens are in the same row, the same column, or on the same diagonal.

Hint: Represent the positions of the queens as a list of numbers 1..N. Example: [4,2,7,3,6,8,5,1] means that the queen in the first column is in row 4, the queen in the second column is in row 2, etc.

type Row a = [a]
type Board a = [Row a]

data State = Queen | NotQueen

type Segment a = [a]

-- Assume that you have this function
-- This function gives you the two diagonals of a board [top left to bottom right, top right to bottom left]:
diags :: Board a -> [Segment a]

Implement the following functions with the eight queens problem in mind:

-- A function that lists all possible combinations of queen positions depending on the number of queens
allQueens :: Int -> [Segment]

So allQueens 8 will give the solution we want.

Computational Complexity

If you haven’t done complexity in class yet, feel free to skip this section and move on to another sheet.

We can apply in-built Haskell functions to the allQueens function. (You can assume the allQueens function is working for this question)

> length (queens 8)
92
> head (queens 8)
[1,5,8,6,3,7,2,4]

What are the complexities of the allQueens, length and head functions respectively?